"Carnot Ratio" claims border Magical properties
The "Carnot Ratio" is universal, temperature determines efficiency, maximum efficiency requires maximum temperatures...
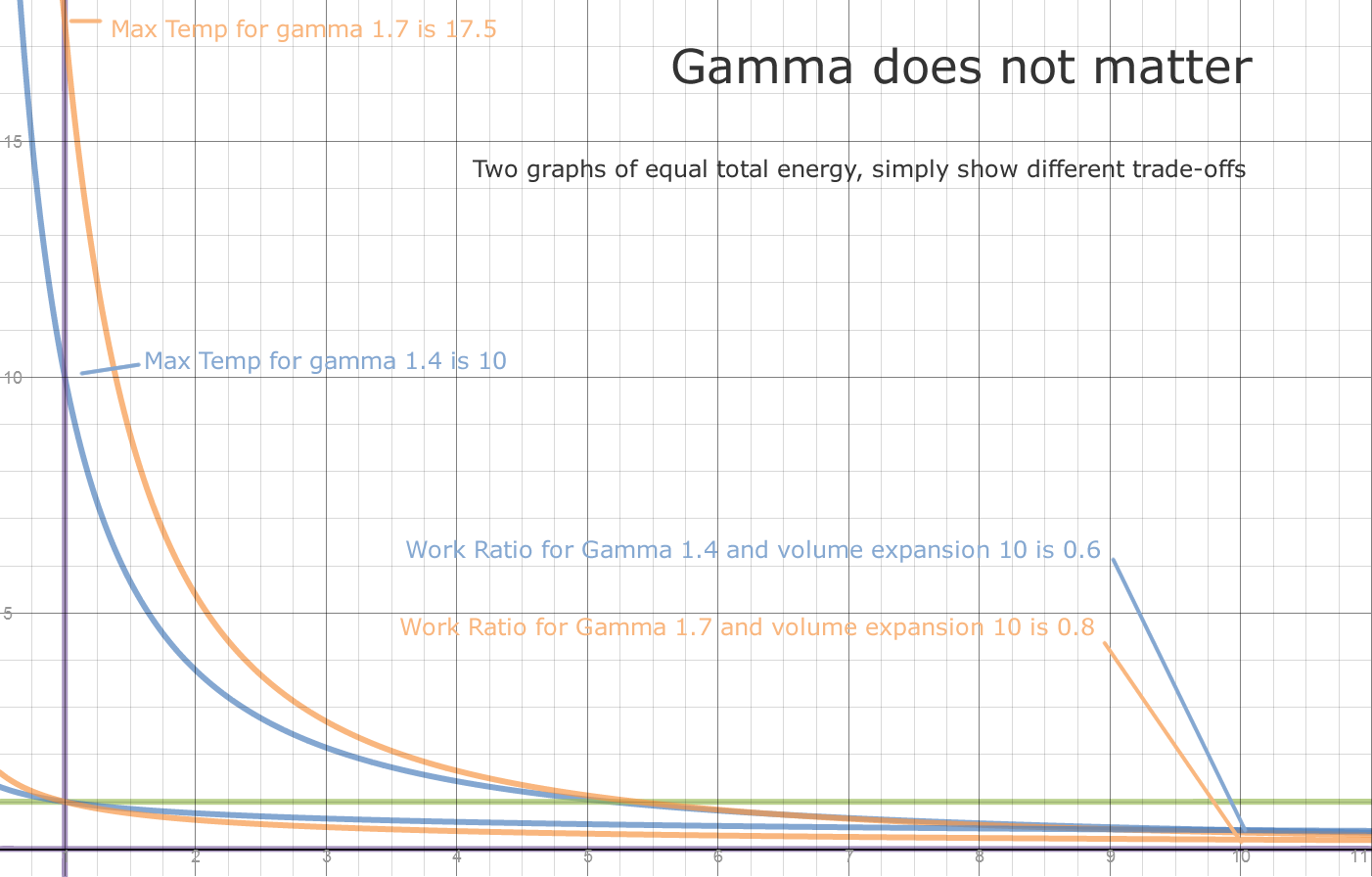
This will get covered in depth later. But its worth mentioning the Carnot ratio for Air and Helium are different, which makes it less than universal by itself. A given energy drop with Helium is a larger temperature drop than for the same Energy drop in Air. Temperature or temperature ratios simply are not directly comparable between materials as a quantity of heat.
If you could make your car engine run on Helium, a 10x expansion ratio would yield a work ratio of .8, instead of Air's work ratio of .6. A car engine at low speeds has a total efficiency of around .07, or an order of magnitude less than the work Ratio. So whatever the issue is with car engines, its not the work ratio.
A Helium Car Engine would have vapor temperatures... almost twice that of air, for the same energy per stroke. Since Internal air temperatures... of car engines already bumps into Steel's melting point, and are well past Aluminum's melting point, material considerations might require reducing the energy per stroke if it could run on Helium.
|
Practical considerations of Gamma
As a practical matter, the energy you get out of a heat engine is the same as the energy you put in, no matter what gamma is.
Even more practical, is to use a cheap, non-polluting, easily available vapor. Air is number 1 in that category, steam a close second.
The shape of the Work curve does not enable or prevent a 100% fuel efficient engine.
|

